This section covers choosing the discard or discards from a tenpai hand that give you the largest wait to win. This entails checking possible waits given a number of slightly different hand makeups.
You should be familiar with an earlier Exam guide, Wait Determination, before you continue, as this guide uses the framework from that page to systematically determine the correct answer for these types of problems.
Tenpai Expansion Method
In this, you pull one tile from each available, quickly do Wait Determination on what is left, and make a note on the result. Once done, the largest wait is your answer. The second number , the number of different tile values you need, is there as a check-back, to help keep count as you calculate the first number.
Example: 













First, set up the Wait Determination array. Above each column, instead of putting the number of the wait, you’ll be putting the number of tiles in that wait (outs) and the number of different waits (types). An example will be given shortly.
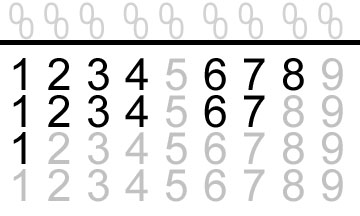
We start by “discarding” a  . This quickly resolves to a 2-sided
. This quickly resolves to a 2-sided 
 wait. there are 4
wait. there are 4  , and 3
, and 3  left, so this is a 7 tile wait, on 2 different tiles: 7-2.
left, so this is a 7 tile wait, on 2 different tiles: 7-2.
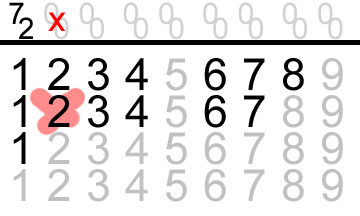
Discarding a  leaves you not tenpai..
leaves you not tenpai..
Same with a  ..
..
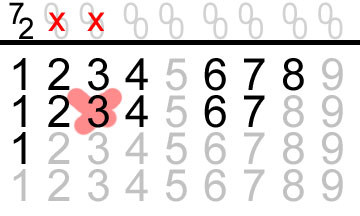
Discarding a  leaves you with the same 2-sided
leaves you with the same 2-sided 
 wait. 7 tiles, 2 types, 7-2.
wait. 7 tiles, 2 types, 7-2.
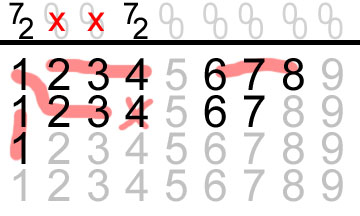
If you find it hard to keep track, you can do a row for each tested tile, to help keep things orderly. (Make your notes from the bottom of the paper so there is room). Here is an example:
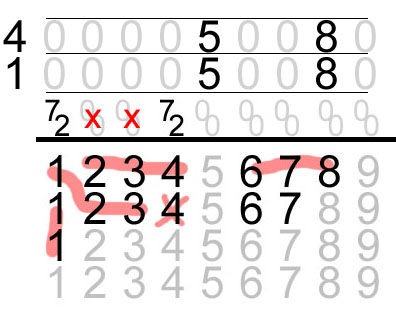
Discarding a  leaves with with a single wait on
leaves with with a single wait on  (the example has it as the pair: you could also have it as a kanchan-style 6_8). There are 2 of them gone, so it is 2 tiles, 1 type, 2-1.
(the example has it as the pair: you could also have it as a kanchan-style 6_8). There are 2 of them gone, so it is 2 tiles, 1 type, 2-1.
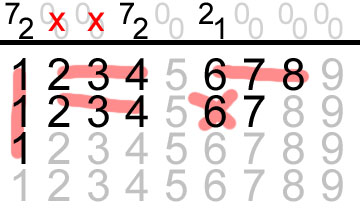
Discarding a  can be seen as 2 different waits: a
can be seen as 2 different waits: a 
 wait, and a
wait, and a  wait. 2 of those 12 tiles are gone, so this is a 10 tile, 3 type wait. 10-3.
wait. 2 of those 12 tiles are gone, so this is a 10 tile, 3 type wait. 10-3.
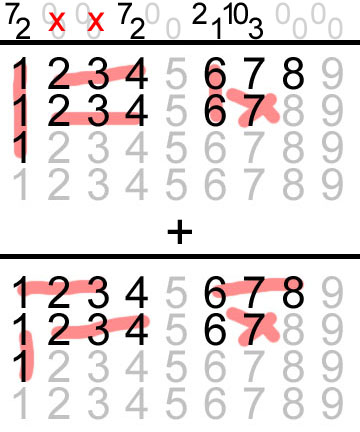
Lastly, discarding an  leaves a double-pair wait,
leaves a double-pair wait, 
 . 4 gone, 4 left, so 4 tiles, 2 types, 4-2.
. 4 gone, 4 left, so 4 tiles, 2 types, 4-2.
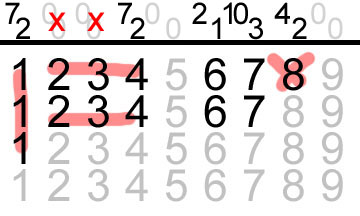
That is all the possible discards. Looking through them, the  wait is the largest, and so the answer is 7p. (If another tile had the same number of “outs” but more “types”, so like 10-4 or 10-5, you would list it in your answer as well)
wait is the largest, and so the answer is 7p. (If another tile had the same number of “outs” but more “types”, so like 10-4 or 10-5, you would list it in your answer as well)
A Warning about Time
Note that this method takes a bit of time. I strongly suggest you leave these sections for last – usually, there are other sections after this on the test with questions of equal value that you can solve much more quickly. Do those first and come back.